Van Der Grinten Projection
Van Der Grinten Projection
Like the globular projection this projection is constructed by drawing circular arcs for both the meridians and the parallels. It has a pleasant balance of shape and scale distortion. The Van der Grinten I projection is a compromise projection and is not in one of the more traditional classifications. It was made famous when the National Geographic Society adopted it as their reference map of the world from 1922 until 1988.

Van Der Grinten Projection Wikipedia
Polar regions are subject to.

Van Der Grinten Projection. This projection is supported in the spherical form only. Van der Grinten 1904 Group. Manifold uses the 1979 formulae published by Snyder.
Minimal distortion along the equator and extreme distortion in the polar regions. Proj4defsESRI53029projvandg lon_00 x_00 y_00 R_A a6371000 b6371000 unitsm no_defs. Limiting Forms Used only in the spherical form.
Van der Grinten published the projection using a geometric construction. Simon Nyvlt ars navigandi See Also. When marked with sizes with and without background are approx.
Van Der Grinten Projection Wikipedia

File Van Der Grinten Projection Of Earth Jpg Wikipedia

Van Der Grinten I Arcgis Pro Documentation
Digital World Map Van Der Grinten Projection Free The World Of Maps Com
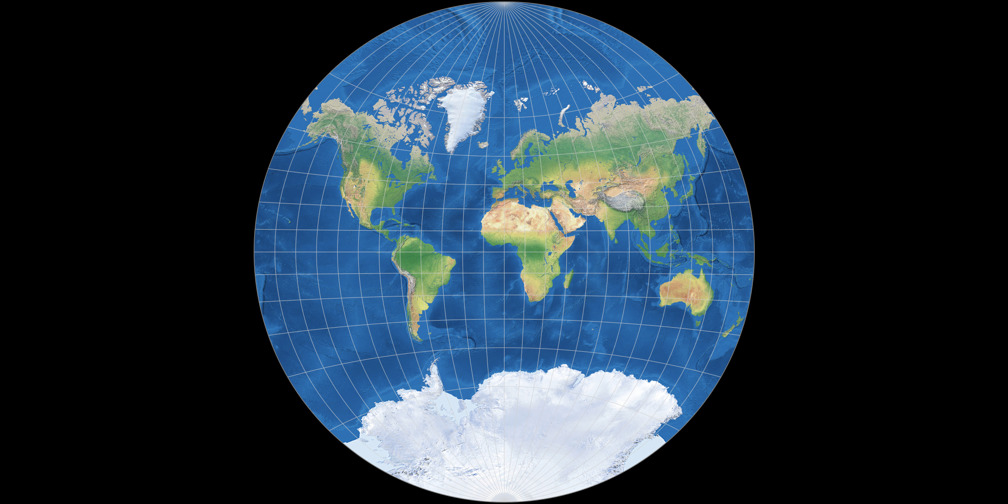
Van Der Grinten I Complete Compare Map Projections

Van Der Grinten Projection Repeating Past 180 Degrees Geographic Information Systems Stack Exchange
Van Der Grinten Iv Compare Map Projections

Beautiful Global Projections Adding Custom Projections To Qgis Free And Open Source Gis Ramblings
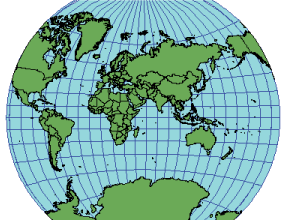
Van Der Grinten I Help Arcgis For Desktop
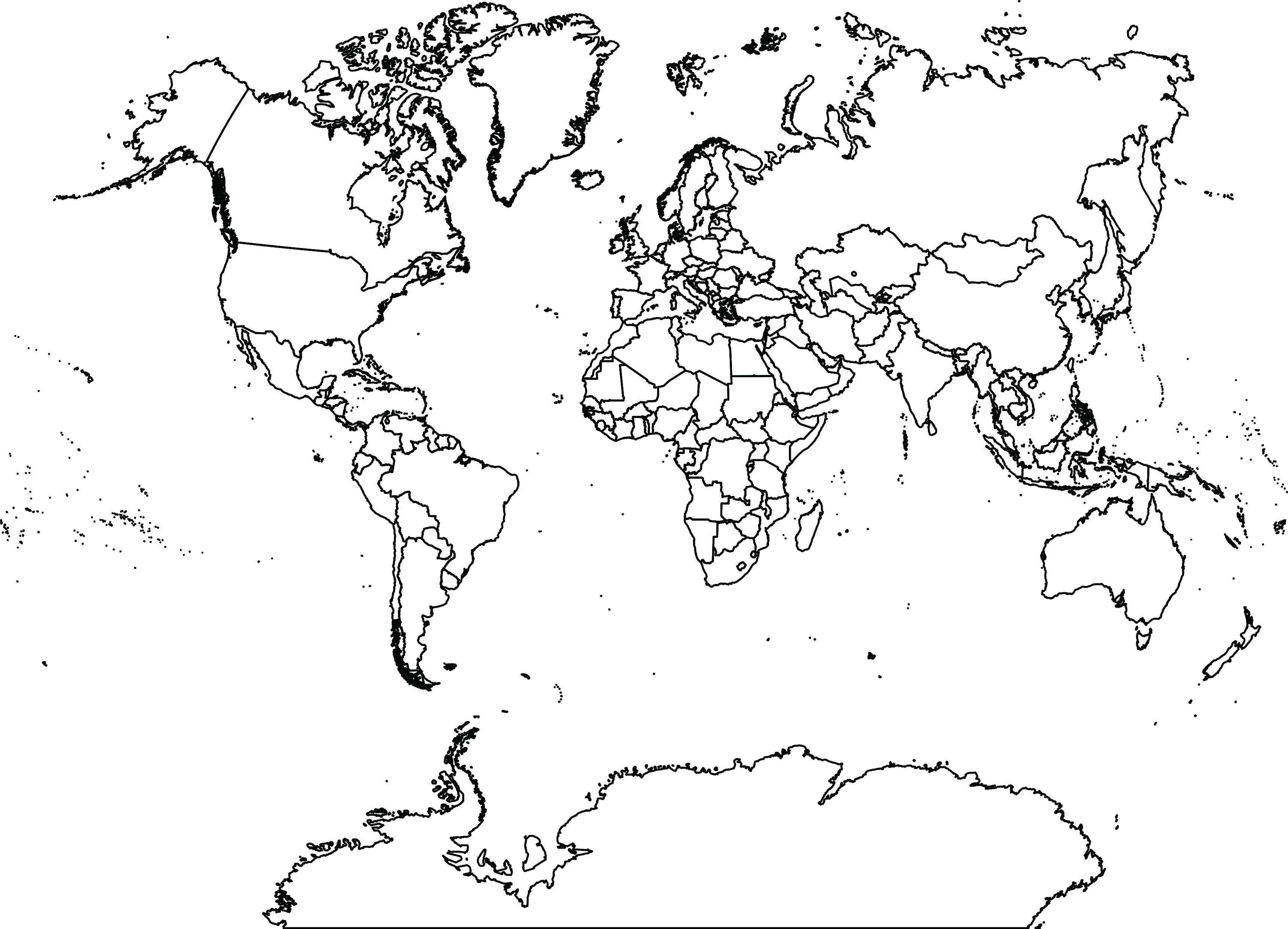
Digital World Map Van Der Grinten Projection Free The World Of Maps Com

World Map Van Der Grinten Projection Stock Vector Illustration Of Chart Outline 7741118
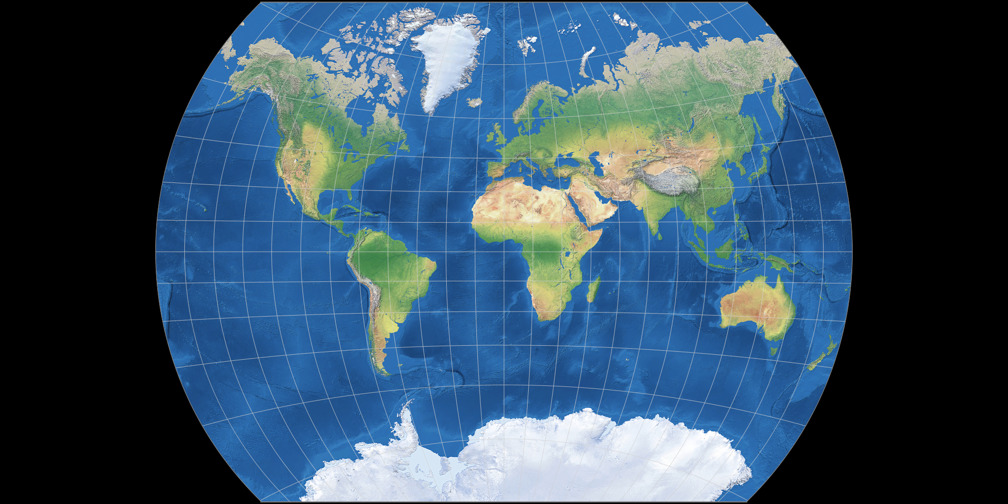
Van Der Grinten I Truncated Compare Map Projections
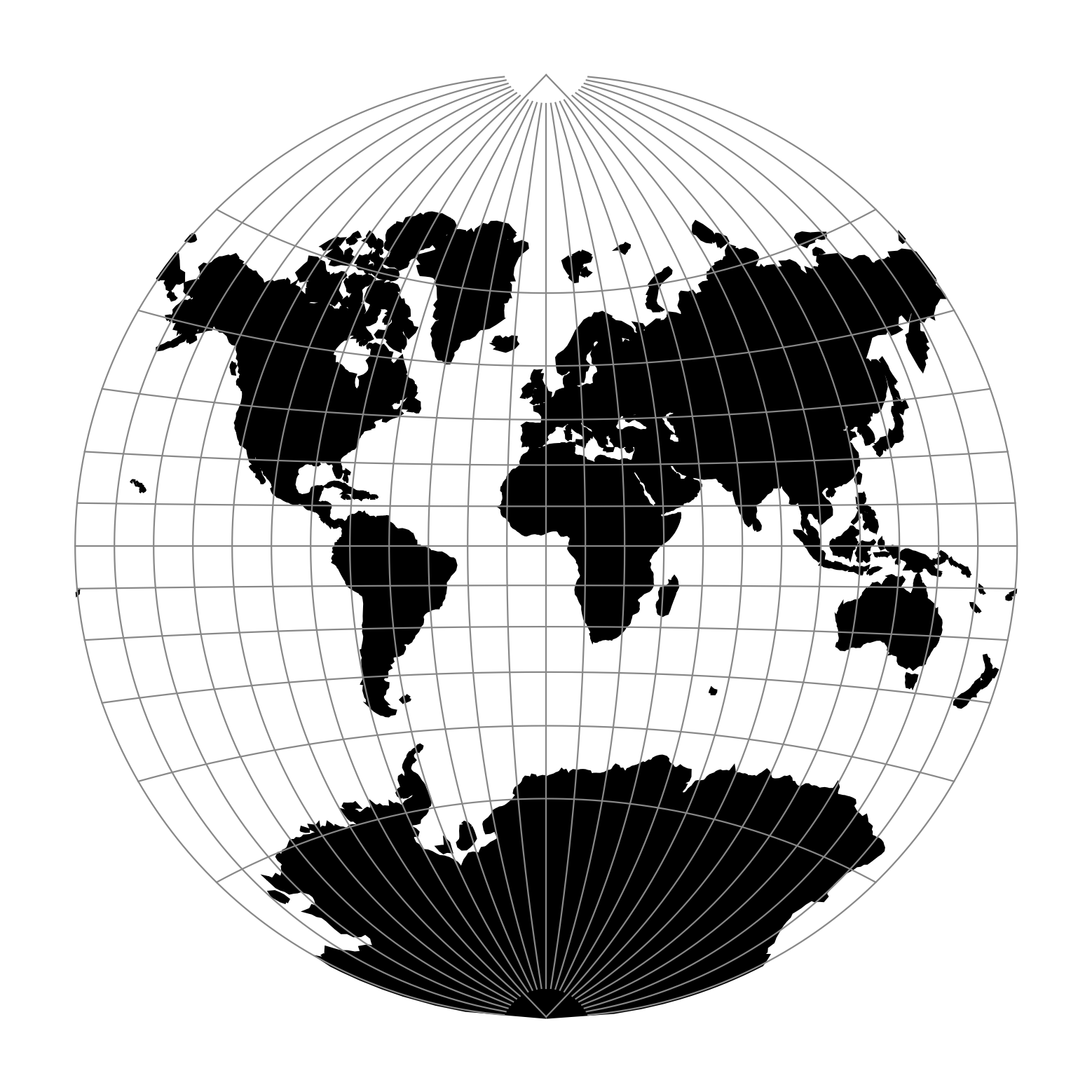
Van Der Grinten I Proj 8 1 0 Documentation

World Map Van Der Grinten Projection Stock Vector Illustration Of Decorative Region 167703065
The Van Der Grinten Projection

Van Der Grinten Projection Basemap Matplotlib Toolkit 1 2 1 Documentation

Van Der Grinten Projection From Wolfram Mathworld


Post a Comment for "Van Der Grinten Projection"